Physics Form One Topic 5: Archimedes’s Principle And The Law Of Flotation - Physics Notes

Welcome to our website darasahuru.co.tz, In this article, Are you looking for Physics Form One Topic 5: Archimedes' Principle And The Law Of Flotation - Physics Notes, Download Physics Notes Form One, New Form One Notes.

Archimedes' Principle

Archimedes' principle states that a body immersed in a fluid is subjected to an upwards force equal to the weight of the displaced fluid.

This is a first condition of equilibrium. We consider that the above force, called force of buoyancy, is located in the centre of the submerged hull that we call centre of buoyancy.

 

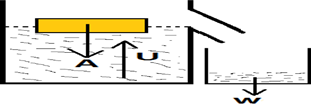
Consider a piece of wood that is held below the surface of a liquid and then released. The wood comes to the surface immediately.

When a piece of wood is immersed in a fluid, then it floats due to the buoyant force or upthrust.

Upthrust is the upward force that enables the object to float or at least seem lighter

The upthrust is greater than the weight of the wood, that is why the wood is pushed to the surface

Archimedes Principle (the law of buoyancy)

It states that:

"When a body is partially or totally immersed in a fluid it experiences an upthrust which is equal to the weight of the fluid displaced"

 

Relationship between Real Weight and Apparent Weight

Consider the diagram of the mass (weight) of the object below

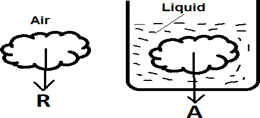
Real Weight is the weight of an object in air

Apparent weight is the weight of an object in fluid

∴ Apparent loss in weight = Real weight – apparent weight of a body in liquid

 

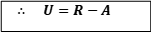
Example

1. Given that the weight of a body in air is 10.10N while the weight of the body when immersed in water is 9.2N. Find the up thrust.

Solution:

Weight in air (R) = 10.10 N Weight in water (A) = 9.2 N

∴

𝑼 = 𝑹 − 𝑨 = 𝟏𝟎. 𝟏𝟎 − 𝟗. 𝟐 = 𝟎. 𝟗 𝑵

Exercise

1. The weight of a body when totally immersed in a liquid is 4.2N if the weight of the liquid displaced is 2.5N. Find the weight of the body in air. (ANS: R =6.7N)

2. When an object is totally immersed in water, its weight is recorded as 3.1N if its weight in air is 4.9N. Find up thrust. (ANS: U = 1.8N)

3. A body immersed in water displaced 1.1N of the liquid if its weight while in water is 3.3N. Find its weight in air. (ANS: R = 4.4N)

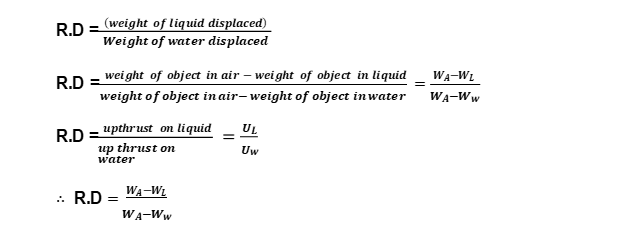
Relative Density by using Archimedes Principle

The relative density of a substance can be expressed as:

Example

1. A piece of glass weighs 5 N in air and 3 N when completely immersed in water calculate its.

(a) Relative density

(b) Density of glass

Solution: Given: R = 5 N

A = 3 N

From:

Relative Density of other liquid from water by solid substance in Archimedes Principle

When a solid immersed in a liquid and water the relative density is given by liquid displaced over water displaced

Mathematically

Example

In an experiment to determine the relative density of a liquid, a solid X weighs as follows:

Weight of X in air, WA (R) = 8.6N Weight of X in water, WA = 6.0N Weight of X in liquid, WL = 5.4N

Solution:

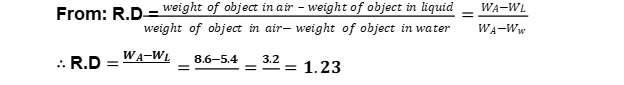
Exercise

1. Using the data shown below and determine the relative density of the liquid

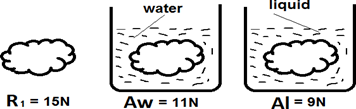
(ANS: R.D = 1.5)

2. A body weighs 0.52 N in air. When total immersed in water it weighs only 0.32N while its weight when immersed in another liquid is 0.36 N. The density of water is 1000 kg/m3. What is the density of the other liquid?

(ANS: ρL = 800 kg/m3)

Sinking

Sinking is the tendency of an object to fall or drop to lower levels in a fluid

 

Conditions for Sinking

The upthrust exerted by the fluid must be less than the weight of an object

The density of the object should be greater than that of the fluid

Floating

Floating is the tendency of an object to remain on the surface of a fluid due to the upthrust.

The ability of an object to float in a fluid is called Buoyancy

Conditions for Floating

The upthrust due to the liquid must be equal to the total weight of the object

The density of the body must be less than that of fluid.

The Volume of submerged object must be large enough to displace a lot of fluid.

Difference between floating and sinking

Floating	Sinking
The body stays at the surface of the liquid	The body drops to the bottom of the liquid
Takes place when the upward force is greater than the weight of the body	Takes place when the upward force is less than the weight of the object
Takes place when the density of the body is less than that of the liquid	Takes place when the density of the body is greater than that of the liquid

The following conditions can be used to determine the position of the object in the fluid: -

If W > U, there is downward movement of the body (known as sinking)

If W < U, there is upward movement of the body

If W = U, the body is in equilibrium under the action of two equal and opposite force. (Thus, the body floats)

The Law of Flotation

It states that:

 

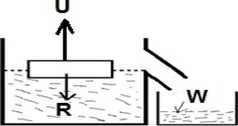
"A floating body displaces its own weight of the fluid in which it floats"

For a substance to float

A. Upthrust (U) = weight of displaced fluid (W)

B. Real weight(R) = weight of displaced fluid (W)

But:

Apparent weight = Real weight – Upthrust (weight of displaced fluid)

For a body to float Apparent weight = 0 N Therefore:    R = U = W

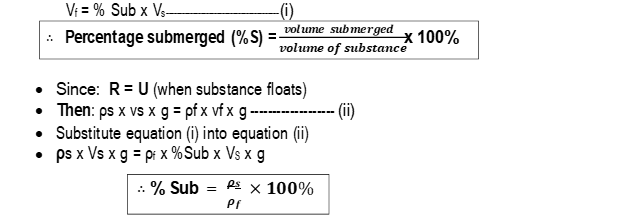
To find percentage of submerged substance consider the equation

Real weight of a substance (R) = Weight of displaced fluid (W)

But now: R = mass of substance (ms) x g

R = density (𝝆s) × volume of substance (vs ) × 𝒈

Also:    U = mass of fluid (mf ) x g = density (𝝆f) × volume of fluid (vf ) × 𝒈

But: Volume of fluid displaced = % of object submerged (S) x Volume of object (Vs)

Application of Flotation

The Law of flotation is applicable in various objects like:-

1. Applied when Filling Balloons (Hot air balloons)

2. Submarines

3. Ships

4. Hydrometer

Balloons (Hot air balloons)

A balloon is a light bag filled with hydrogen or helium gas.

These gases are less dense than air. An air ship is a large balloon with a motor to make it and fins to steer it.

The downward force in a balloon is equal to the weight of the bag plus the weight of a gas in it.

 

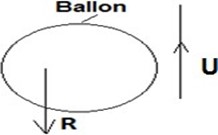
The balloon rises if the upthrust is greater than the down ward force. That is

NB:

As a balloon rises, the atmospheric pressure on it becomes less. The gas in the balloon tends to expands

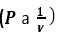
Therefore, the gas – bag must not

be filled completely when the balloon is on the ground.

Consider the diagram below

If the balloon is filled with some gas of known density. Then the volume of gas required just to lift the balloon into the air is given by

Example

1. A balloon and the gas in it has a mass of 450 g and its volume is 500 cm3. What is the maximum load it can lift in air of density 1.3 g/cm3

Solution:

From: The Principle of floatation,

Mass of balloon + Load = Mass of air displaced

= Volume of gas x density of air

= 500 x 1.3 = 650 g

Exercise

1. A hot air balloon including the envelope, gondola, burner and fuel and one passenger has a total mass of 450kg. Air outside balloon is at 20℃ and has a density of 1.29kg/m3 the air inside at temperature 120℃ has density of 0.90kg/m3. To what volume must the envelope expand to just lift the balloon into the air? (ANS: V= 1154 m3)

2. A balloon has a capacity of 20m3 and it is filled with hydrogen. The balloon fabric and the container have a mass of 2.5kg. What mass of instruments can be lifted by the balloon? (Density of hydrogen = 0.089kg/m3 and density of air is 1.29kg/m3) (ANS: m = 21.52kg)

Sub Marines

A Submarine is a watercraft capable of independent operation underwater

A submarine has ballast tanks which can be filled with water or air

When full of water ,the average density of the submarine is slightly greater than the density of the sea – water and it sinks

When air is pumped into the tanks the average density of the submarine falls until it is the same or slightly less than that of the water around it

The submarine therefore stays at one depth or rises to the surface

Ships

A ship is a large watercraft that travels the world's oceans and other sufficiently deep waterways

It is used to carry passengers or goods or in supporting specialized missions such as defense, research and fishing

A Ship is made of steel and is expected to sink due to its weight. it contains hollow which increases the volume of ship which helps on making less denser than water

So for safety loading of the ship under different sea conditions plimsol lines (plimsol marks) are provided

Plimsoll lines: Are lines which show maximum height of the ship that should be under water

  

Plimsoll lines are also referred as plimsol marks. See the figure below

Whereby:

F =	Fresh water	S =	Summer sea
W =	Winter Sea	TF =	Tropical fresh water
WNA =	Winter North Atlantic	T    =	Tropical seas

Hydrometer

Is a floating instrument used for measuring the densities of liquids.  OR

  

Is an instrument used for determining the relative density of liquids.

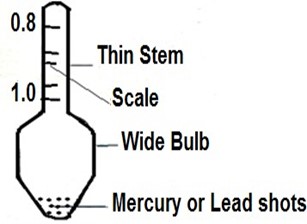
Parts (Structure) of Hydrometer

Heavy sinker (bulb): containing mercury or lead shots that keeps the hydrometer upright when it floats

Air bulb: it increases volume of displaced liquid and overcomes the weight of the sinker

Stem: stem is thin so that small changes in density (height) gives large difference in reading

Scale: Inside stem graduated in densities

It is made up of glass to prevent soaking of the liquid

Mode of action of hydrometer

A hydrometer is made to float in the liquid whose relative density is to be measured

It sinks to different levels depending on the relative density of the liquid in which it floats

The liquid whose relative density is to be determined is poured into a tall jar and the hydrometer is gently lowered into the liquid until it floats freely.

The point where the surface of the liquid touches the stem of the hydrometer indicates the relative density of the liquid

Example, the hydrometer sinks more in methanol than in water. This indicates that water is denser than methanol

NB:

The greater the density of the liquid the shorter the stem of hydrometer immersed

Hydrometer works on the principle of Archimedes

Relative Density of Liquid by Hydrometer

When hydrometer floats over water the weight of hydrometer (wh) must be equal to the weight of water displaced (ww)    That is wh = ww

When hydrometer floats over liquid the weight of hydrometer (wh) must be equal to the weight of liquid displaced (wL)    That is wh = wL

Since the relative density of liquid is given by ratio of density of liquid (ρL) to the density of water (ρw)

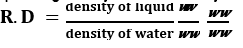
BUT

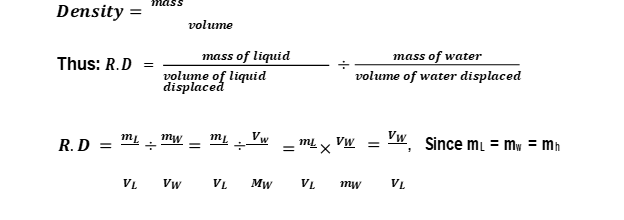
Since cross-section area of the hydrometer is uniform, the volume of water and that of liquid displaced are proportional to the lengths immersed in them

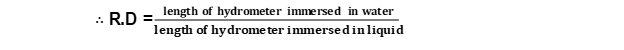
But: R = U (Weight of hydrometer = Up thrust of liquid)

Consider the diagram below

Whereby:

Stem volume, V1 = Ah

Bulb volume,    V2 = V

Total volume, VT = V1 + V2 = Ah + V

Whereby:

ρmn = minimum density ρmx = maximum density U = Vt x ρmn x g

R = V x ρmx x g

Then:

Vt x ρmn x g = V x ρmx x g

(Ah + V) x ρmn x g = V x ρmx x g (Ah + V) x ρmn = V x ρmx

Ah x ρmn + V x ρmn = V x ρmx    make V2 the subject

V x ρmx – V x ρmn = Ah x ρmn V x (ρmx – ρmn) = Ah x ρmn

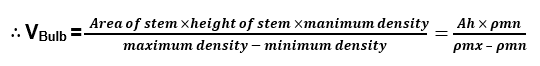
Examples

1. Consider the diagram below used to measure density of liquid between 1g/cm3 to 0.81g/cm3 (The area of cross section area of stem is 0.5 cm2). Find the volume of hydrometer below 1.0 g/cm3 graduated

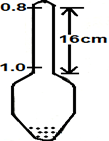
Data given

Cross section area of stem, A = 0.5cm2 Height of steam, h = 16 cm

The volume of steam, V1 = Ah = 8 cm3 Total volume, VT = (8 + V2) cm3

Minimum density, ρmn = 0.8 g/cm3 Maximum density, ρmx = 1.0 g/cm3 The volume of bulb, V2 =?

Solution

From

2. The diagram below shows on form of man hydrometer used to measure the densities of liquid over the range of 0.8 to 1.00 g/cm3. If the area of cross section of the stem is 0.5 cm2 and the distance between the 0.80 and 100 division is 18cm determine

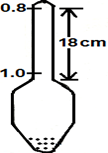
a). The volume of hydrometer below 1.00 graduated

b). The position of the 0.90 graduation

Solution:

Given Cross section area of stem, A = 0.5cm2 Height of stem, h = 18 cm

The volume of stem, V1 = Ah = 9 cm3

Total volume, Vt = (9 + V2) cm3 Minimum density, ρmn = 0.8 g/cm3 Maximum density, ρmx = 1.0 g/cm3 The volume of bulb, V2 =?

a) The volume of bulb, V2 = ?

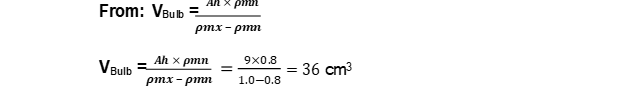
b) What height, h2 of hydrometer when shifted to measure 0.9 g/cm3

From

Daily Application of the Law of Floatation

1. It is used in transportation by water ways: (By ships, submarines and ferry boats)

2. It is used in transportation by air ways:(By hot air balloons and air ships)

3. It is used in decoration: (Balloons of different colors and shapes are filled with lighter gas so that will float in air)

4. It is used in determination of specific gravity of liquids: (By hydrometer)

Assignment

1. (a) Why does a solid body weigh more in air than when immersed in a liquid?          

    (b)An ordinary hydrometer of mass 27g floats with 4cm of its stem out of water.

If the cross-section area of the stem is 0.75cm2. Calculate

i) The total volume of stem just under the surface of the liquid (ANS: V = 30 cm3)

ii) The relative density of the liquid (ANS: R.D = 0.9)

2. A balloon of volume 2000 m3 is filled with hydrogen of density 0.09 kg/m3. If the mass of fabric is 100kg and that of the pilot is 75kg,

i) What will be the greatest mass of equipment that can be carried when operation in air is 1.25kg/m3?    (ANS: m =2145 kg)

ii) How would this figure change if helium, which has twice the density of hydrogen under the same condition, were to be used?(AN: m=1965 kg)

3. The mass of a piece of cork (0.25 g/cm3) is 20g. What fraction of the cork is immersed when it floats in alcohol ?(density of alcohol is 0.8 g/cm3) (𝑨𝑵𝑺: 𝟓 )

4. A uniform pencil floats upright in water with 8cm of its length immersed. What length is immersed when it floats in glycerol (density of glycerol is 1.3 g/cm3)?

(ANS: L = 6.2 cm)

5. A balloon and the gas in it has a mass of 500 g and its volume is 600 litres. What is the maximum load it can lift in air of density 1.3 g/dm3? (ANS: m=280 g)